acolz.blogg.se - Depriester chart to read boiling point

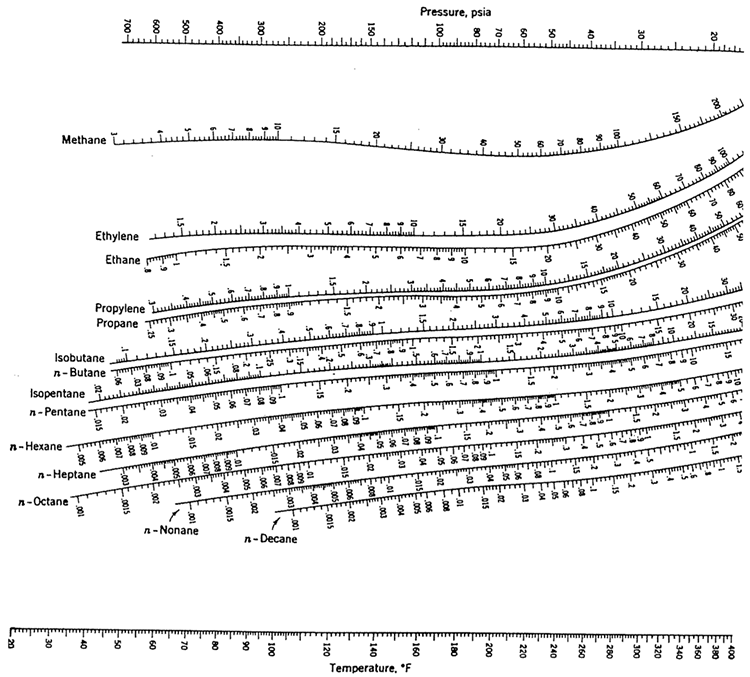
Plot Tx1y1 at P=70kPaChoose T between T1sat and T2sat, calculate P1sat and P2sat and use these to calculate x1 by the following eqn. =1 (10.14) If Raoult's Law valid, Pd 1yiPi So lets calculate Pd at z1=0.6 and T=75oC.This is point c in previous Px1y1 diagram. For Raoult's Law, P xiPisat (10.2) P x1P1sat x2P2sat x1P1sat (1 x1)P2satP (P1sat P2sat )x1 P2sat note: a linear line (y=mx+c)Ĭalculate P for a set of x1 and then calculate y1using,ġ83.211750.888074.960.8750.748366.720.6750.569258.470.4750.331350.230.275041.98075y1P(kPa)x1Tī sat liquid solution or bubblepointPb is by BUBL PĬ sat vapor mixtureor dewpointPd is by DEW PĭEW P calculationCalculate Pd and x1, given y1 and T Substitute yi Kixi into (A)zi (1V )xi KixiV xi (1V VKi ) xi (1V (Ki 1))xiĪcetonitrile(1)/Nitromethane(2)Antoine Eqn,Ĭalculate P and y1, given a set of x1 and T=75oCThis is BUBL P calculation. Let T=1 mol, so V and L are mole fractionszi Lxi Vyi zi (1V )xi Vyi (A) To calculate the T when the last dew disappear as a result of increase in T at constant P. To calculate the T when the 1st dew (drop of liquid) appear as a result of decrease in T at constant P Read value of K-valueat given T and Pe.g.

For Raoults law, needs PisatTherefore requires Tįor light hydrocarbon mixture where Kiis essentially function of T and P only, the valuecould be simply tabulated as in DePriester chart.

Most chemical processes operate at this P, T rangeĪzeotropex1=y1 x2=y2Liquid composition= Gas composition Note: species 1 has higher vapor pressure or lower boiling point than species 2

LV = tie lineL = bubble pointW = dew point KJIHLK givesFigure 10.2 (b)Txy phase diagram at constant Pį (liquid solution)L (bubble point)W (dew point)G (Gas mixture) Top surface issaturated-liquid(P,T,x1 surface)ĪEDBLA givesFigure 10.2 (a)Pxy phase diagram at constant T Under surface issaturated-vapor (P,T,y1 surface) T curve for species 2Ĭ1 and C2 are critical points for species 1 & 2

Carry out bubble and dew point calculations for a given mixture Carry out flash calculation in order to determine the vapor/liquidįraction as well as the mixture composition of each phase at specified conditions using available K-Values etc. Apply Raoults law and Henrys law to solve simple thermodynamic

Apply simplified VLE equations to obtain data for P-XY, T-XY and X. It is expected that students have the ability to: Describe the behaviour of VLE and how to simplify the VLE problem. SKF 2213 Chemical Engineering Thermodynamics